Line Integrals

In single variable calculus, we have definite integrals

$${\int }_{x=a}^{b}f(x)dx$$

We integrate f(x) along a straight line

Note: If f(x) =1

$${\int }_a^{b}dx=x{|}_a^b=b-a\Rightarrow \text{length of line}$$

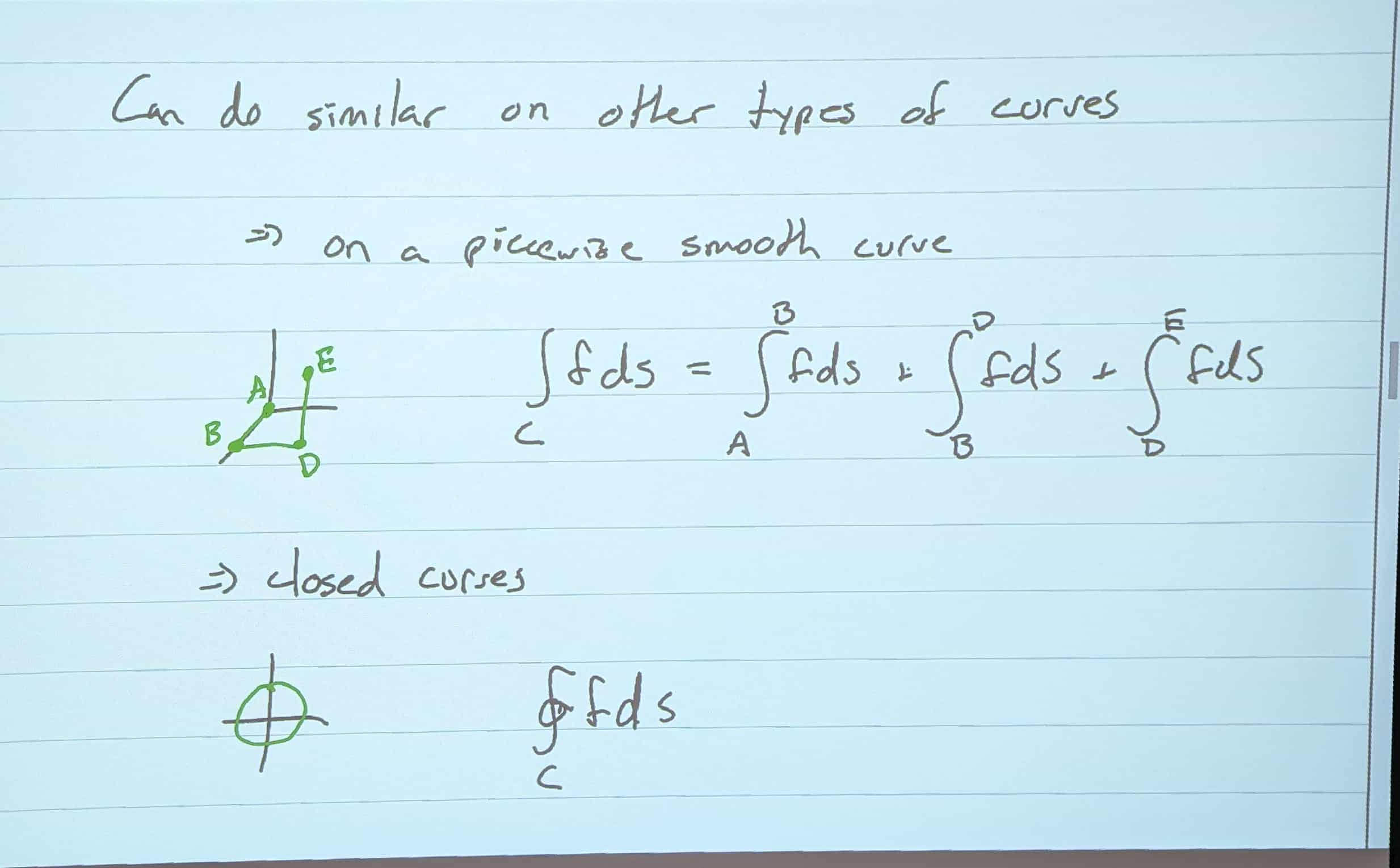
Now: Curve r(t) = (x(t), y(t), z(t))

$${\int }_{t=a}^{b}ds=\text{arclength}$$

This is a special case of “Line Integrals”

In 3D:

$${\int }_{t=a}^{b}f(x,y,z)ds$$

In 2D:

$${\int }_{t=a}^{b}f(x,y)ds$$

If (x,y,z) = 1, we have an arclength

How do we solve this?

$${\int }_{c}fds\text{integrating f over a curve c}$$

We need to transform this into a definite integral!

Step 1: Find a parametric description of c

$$\vec{r}(t)=x(t),y(t),z(t)$$

Step 2: Find f(x,y,z) on r(t) We plug in and get f(t)

Step 3: Find ds in terms of dt Recall that: $ds=||\vec{v}||dt=||\frac{d\vec{t}}{dt}||dt$ ${\int }_{t=a}^{b}f(t)||\frac{d\vec{t}}{dt}||dt$

Here’s an abridged example, abbreviated as we did part of it yesterday. We plug in r(t).

$$\oint \Leftarrow \text{This means that the curve is closed! It is treated the same}$$

Line Integrals and Vector Fields

Work

Work is a measure of F in the tangent direction!

$$\vec{F}\cdot \hat{T}$$ $$\text{Work}={\int }_c\vec{f}\cdot \hat{T}ds$$

OR

$$\text{Work}={\int }_c\vec{f}\cdot \frac{d\vec{r}}{dt}dt$$

Flux

Work is a measure of F in normal direction! Think of this as the flow across the curve.

$$\vec{F}\cdot \hat{N}$$

Remember, now N = T x K

We’ll also say for flux, f(t) = (P,Q) instead of X and Y

$$\text{Flux}={\int }_c\vec{f}\cdot \hat{N}ds$$

OR

$$\text{Flux}={\int }_c(P\frac{dy}{dt}-Q\frac{dx}{dt})dt$$

Takeaways: Work = Tangential Direction, Flux = Normal Direction

---

Line Integrals and Special Fields

If F is conservative, ∇ x f =0

If F is incompressible, ∇ ⋅ f =0